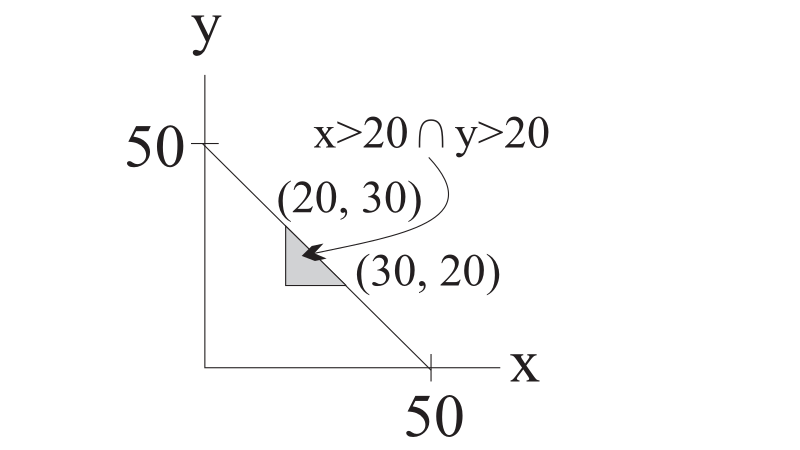The future lifetimes (in months) of two components of a machine have the following joint density function:

[[math]] f(x,y) = \begin{cases} \frac{6(50-x-y)}{125000}, \,\, 0 \lt x \lt 50-y \lt 50 \\ 0, \, \textrm{Otherwise.} \end{cases} [[/math]]

Determine which of the following represents the probability that both components are still functioning 20 months from now.

• [math]\frac{6}{125000}\int_{0}^{20}\int_{0}^{20}(50-x-y)\,ds\,dt[/math]
• [math]\frac{6}{125000}\int_{20}^{30}\int_{20}^{50-x}(50-x-y)\,ds\,dt[/math]
• [math]\frac{6}{125000}\int_{20}^{30}\int_{20}^{50-x-y}(50-x-y)\,ds\,dt[/math]
• [math]\frac{6}{125000}\int_{20}^{50}\int_{20}^{50-x}(50-x-y)\,ds\,dt [/math]
• [math]\frac{6}{125000}\int_{20}^{50}\int_{20}^{50-x-y}(50-x-y)\,ds\,dt [/math]

Copyright 2023. The Society of Actuaries, Schaumburg, Illinois. Reproduced with permission.

Solution: B

In order to determine integration limits, consider the following diagram:

We conclude that

[[math]] \operatorname{P}[X \gt 20 \cap Y \gt 20] = \frac{6}{125000} \int_{20}^{30} \int_{20}^{50-x} (50-x-y) dy dx. [[/math]]

Copyright 2023. The Society of Actuaries, Schaumburg, Illinois. Reproduced with permission.