exercise:10d60b4a8e: Difference between revisions
From Stochiki
(Created page with "<div class="d-none"><math> \newcommand{\NA}{{\rm NA}} \newcommand{\mat}[1]{{\bf#1}} \newcommand{\exref}[1]{\ref{##1}} \newcommand{\secstoprocess}{\all} \newcommand{\NA}{{\rm NA}} \newcommand{\mathds}{\mathbb}</math></div> <ul><li> There has been a blizzard and Helen is trying to drive from Woodstock to Tunbridge, which are connected like the top graph in Figure \ref{fig 4.51}. Here <math>p</math> and <math>q</math> are the probabilities that the two roads are pass...") |
No edit summary |
||
| Line 6: | Line 6: | ||
\newcommand{\NA}{{\rm NA}} | \newcommand{\NA}{{\rm NA}} | ||
\newcommand{\mathds}{\mathbb}</math></div> | \newcommand{\mathds}{\mathbb}</math></div> | ||
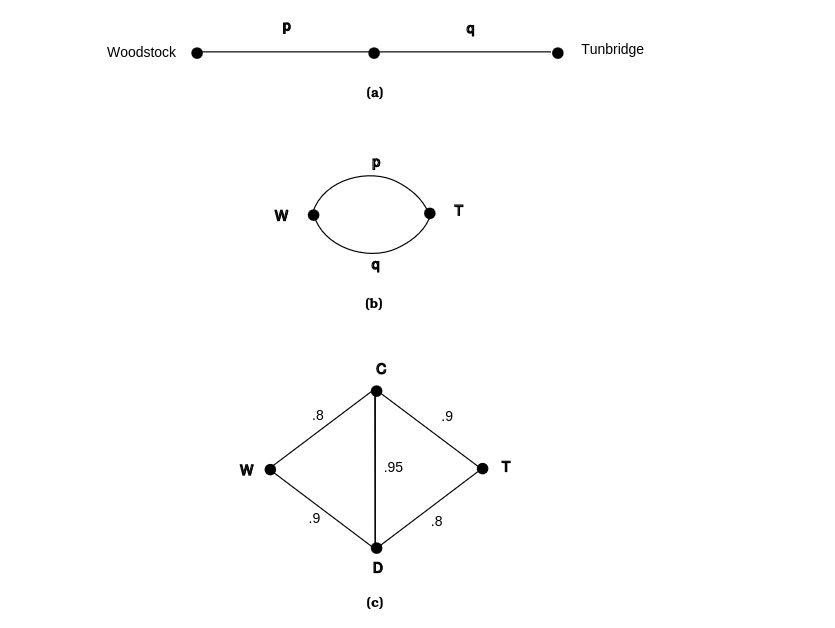
<ul><li> There has been a blizzard and Helen is trying to drive from Woodstock | <ul style="list-style-type:lower-alpha"><li> There has been a blizzard and Helen is trying to drive from Woodstock | ||
to Tunbridge, which are connected like the top graph in | to Tunbridge, which are connected like the top graph in [[#fig 4.51|Figure]]. Here <math>p</math> and <math>q</math> are the probabilities that the two roads are passable. What is the probability that Helen can get from Woodstock to Tunbridge? | ||
4.51 | |||
is the probability that Helen can get from Woodstock to Tunbridge? | |||
</li> | </li> | ||
<li> Now suppose that Woodstock and Tunbridge are connected like the middle graph | <li> Now suppose that Woodstock and Tunbridge are connected like the middle graph | ||
in | in [[#fig 4.51|Figure]]. | ||
What now is the probability that she can get from <math>W</math> to <math>T</math>? Note that if we | What now is the probability that she can get from <math>W</math> to <math>T</math>? Note that if we think of the roads as being components of a system, then in (a) and (b) we have computed the ''reliability'' of a system whose components are (a) ''in series'' and (b) ''in parallel.'' | ||
think of the roads as being components of a system, then in | |||
(a) and (b) we have computed the | |||
''reliability'' of a system whose components are (a) ''in | |||
series'' and (b) ''in parallel.'' | |||
</li> | </li> | ||
<li> Now suppose <math>W</math> and <math>T</math> are connected like the bottom graph in | <li> Now suppose <math>W</math> and <math>T</math> are connected like the bottom graph in [[#fig 4.51|Figure]]. | ||
Find the probability of Helen's getting from <math>W</math> to <math>T</math>. '' Hint'': If the | Find the probability of Helen's getting from <math>W</math> to <math>T</math>. '' Hint'': If the road from <math>C</math> to <math>D</math> is impassable, it might as well not be there at all; if it is passable, then figure out how to use part (b) twice. | ||
road from <math>C</math> to <math>D</math> is impassable, it might as well not be there at all; if it | |||
is passable, then figure out how to use part (b) twice. | |||
</li> | </li> | ||
</ul> | </ul> | ||
<div id=" | <div id="fig 4.51" class="d-flex justify-content-center"> | ||
[[File:guide_e6d15_PSfig4. | [[File:guide_e6d15_PSfig4.png | 600px | thumb | From Woodstock to Tunbridge. ]] | ||
</div> | </div> | ||
Latest revision as of 16:24, 19 June 2024
[math]
\newcommand{\NA}{{\rm NA}}
\newcommand{\mat}[1]{{\bf#1}}
\newcommand{\exref}[1]{\ref{##1}}
\newcommand{\secstoprocess}{\all}
\newcommand{\NA}{{\rm NA}}
\newcommand{\mathds}{\mathbb}[/math]
- There has been a blizzard and Helen is trying to drive from Woodstock to Tunbridge, which are connected like the top graph in Figure. Here [math]p[/math] and [math]q[/math] are the probabilities that the two roads are passable. What is the probability that Helen can get from Woodstock to Tunbridge?
- Now suppose that Woodstock and Tunbridge are connected like the middle graph in Figure. What now is the probability that she can get from [math]W[/math] to [math]T[/math]? Note that if we think of the roads as being components of a system, then in (a) and (b) we have computed the reliability of a system whose components are (a) in series and (b) in parallel.
- Now suppose [math]W[/math] and [math]T[/math] are connected like the bottom graph in Figure. Find the probability of Helen's getting from [math]W[/math] to [math]T[/math]. Hint: If the road from [math]C[/math] to [math]D[/math] is impassable, it might as well not be there at all; if it is passable, then figure out how to use part (b) twice.